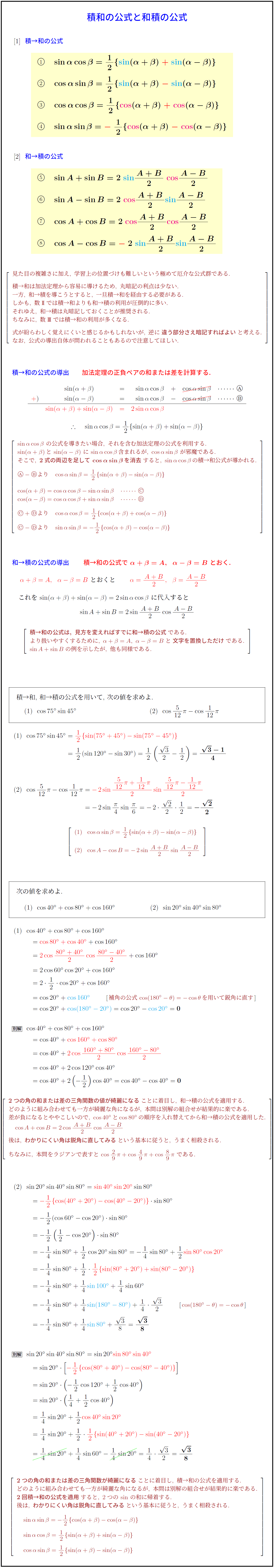
積→和の公式
① $\sinα\cosβ=12\{\sin}(α+β)\ +}\ \sin}(α-β)\$}
② $\cosα\sinβ=12\{\sin}(α+β)\ -}\ \sin}(α-β)\$}
③ $\cosα\cosβ=12\{\cos}(α+β)\ +}\ \cos}(α-β)\$}
④ $\sinα\sinβ=-}\ 12\{\cos}(α+β)\ -}\ \cos}(α-β)\和→積の公式
⑤ $\sin A+\sin B=2\ \sin}A+B}{2}\ \cos}A-B}{2$}
⑥ $\sin A-\sin B=2\ \cos}A+B}{2}\sin}A-B}{2$}
⑦ $\cos A+\cos B=2\ \cos}A+B}{2}\cos}A-B}{2$}
⑧ $\cos A-\cos B=-}\ 2\ \sin}A+B}{2}\sin}A-B}{2$}
\end{tabular
見た目の複雑さに加え,\ 学習上の位置づけも難しいという極めて厄介な公式群である.
積→和は加法定理から容易に導けるため,\ 丸暗記の利点は少ない.
一方,\ 和→積を導こうとすると,\ 一旦積→和を経由する必要がある.
しかも,\ 数I-.2zw}I}では積→和よりも和→積の利用のほうが多い.
それゆえ,\ 和→積は丸暗記しておくことが推奨される.
ちなみに,\ 数I-.2zw}I-.2zw}I}では積→和の利用が多くなる.
式が紛らわしく覚えにくいと感じるかもしれないが,\ 逆に違う部分さえ暗記すればよい}と考える.
なお,\ 公式の導出自体が問われることもあるので注意してほしい.
積→和の公式の導出 加法定理の正負ペアの和または差を計算する.
$cccrclc}
& \sin(α+β)&=&\sinα\cosβ&+&\teisei{\cosα\sinβ & ・・・・・・\,\maru{ A} \\
+}) & \sin(α-β)&=&\sinα\cosβ&-&\teisei{\cosα\sinβ& ・・・・・・\,\maru{ B} \\ \hline
& \sin(α+β)+\sin(α-β)} & =} & 2\sinα\cosβ}
\end{array}$} \\
∴ \sinα\cosβ=12\{\sin(α+β)+\sin(α-β)\}$}
\sinα\cosβ\ の公式を導きたい場合,\ それを含む加法定理の公式を利用する.
\sin(α+β)\,と\,\sin(α-β)\ に\,\sinα\cosβ\,含まれるが,\ \cosα\sinβ\ が邪魔である.
そこで,\ 2式の両辺を足して\,\cosα\sinβ\,を消去}すると,\ \sinα\cosβ\,の積→和公式が導かれる.
\maru{ A}-\maru{ B}より \cosα\sinβ=12\{\sin(α+β)-\sin(α-β)\}
\cos(α+β)=\cosα\cosβ-\sinα\sinβ ・・・・・・\,\maru{ C}
\cos(α-β)=\cosα\cosβ+\sinα\sinβ ・・・・・・\,\maru{ D}
\maru{ C}+\maru{ D}より \cosα\cosβ=12\{\cos(α+β)+\cos(α-β)\}
\maru{ C}-\maru{ D}より \sinα\sinβ=-12\{\cos(α+β)-\cos(α-β)\}
\end{array\right]$ \\
和→積の公式の導出 $積→和の公式で\ α+β=A,\ \ α-β=B\ とおく.$
$α+β=A,\ \ α-β=B}\ とおくと α=A+B}{2},\ \ β=A-B}{2$
これを $\sin(α+β)+\sin(α-β)=2\sinα\cosβ\ に代入すると$
$\sin A+\sin B=2\sinA+B}{2}\cosA-B}{2}$}
積→和の公式は,\ 見方を変えればすでに和→積の公式}である.
より扱いやすくするために,\ α+β=A,\ α-β=Bと文字を置換しただけ}である.
\sin A+\sin Bの例を示したが,\ 他も同様である.
積→和,\ 和→積の公式を用いて,\ 次の値を求めよ.
(1)\ \ $\cos75°\sin45°$ (2)\ \ $\cos5}{12}π-\cos1}{12}π$ \\
(1)\ \ $\cos75°\sin45°=12\{\sin(75°+45°)-\sin(75°-45°)\$
$\cos75°\sin45°}=12(\sin120°-\sin30°)=12√3}{2}-12=√3-1}{4$
(2)\ \ $\cos5}{12}π-\cos1}{12}π=-\,2\sin5}{12}π+1}{12}π}{2}\sin5}{12}π-1}{12}π}{2$
$\cos5}{12}π-\cos1}{12}π}=-\,2\sinπ}{4}\sinπ}{6}=-\,2・√2}{2}・12=-√2}{2$ \\
$\left[l}
(1)\ \ \cosα\sinβ=12\{\sin(α+β)-\sin(α-β)\}
(2)\ \ \cos A-\cos B=-\,2\sinA+B}{2}\sinA-B}{2}
次の値を求めよ. $\cos40°+\cos80°+\cos160°$ (2)\ \ $\sin20°\sin40°\sin80°$ \\
2つの角の和または差の三角関数の値が綺麗になる}ことに着目し,\ 和→積の公式を適用}する.
どのように組み合わせても一方が綺麗な角になるが,\ 本問は別解の組合せが結果的に楽である.
差が負になるとややこしいので,\ \cos40°\,と\cos80°\,の順序を入れ替えてから和→積の公式を適用した.
\cos A+\cos B=2\cosA+B}{2}\cosA-B}{2}
後は,\ わかりにくい角は鋭角に直してみる}という基本に従うと,\ うまく相殺される.
ちなみに,\ 本問をラジアンで表すと\,\cos29π+\cos49π+\cos89π\ である.
2つの角の和または差の三角関数が綺麗になる}ことに着目し,\ 積→和の公式を適用}する.
どのように組み合わせても一方が綺麗な角になるが,\ 本問は別解の組合せが結果的に楽である.
2回積→和の公式を適用}すると,\ 2つの\,\sin\,の和に帰着する.
後は,\ わかりにくい角は鋭角に直してみる}という基本に従うと,\ うまく相殺される.
\sinα\sinβ=-12\{\cos(α+β)-\cos(α-β)\}
\sinα\cosβ=12\{\sin(α+β)+\sin(α-β)\}
\cosα\sinβ=12\{\sin(α+β)-\sin(α-β)\}

